Quantum Computing: Hype or Reality?

Quantum computing is a field that has garnered a lot of attention in recent times. Some believe that it is the next industrial revolution that will change the world, while others believe that it is all hype and that quantum computers are interesting but useless. A new paper published by scientists has added to the latter camp, raising doubts about the practicality of quantum computing.

Quantum computers are devices that exploit quantum links, known as entanglement. These devices can perform some calculations much faster than conventional computers because they have additional ways to encode and handle information. However, not all calculations can be performed faster by quantum computers. Nevertheless, the calculations that quantum computers can speed up cover several commercially relevant cases, such as quantum chemistry, logistics, and finance.

Despite the potential of quantum computing, there are many practical problems in making quantum computers work. For one, quantum links are incredibly fragile and last only a few microseconds to a few seconds, depending on how they are created. Additionally, the quantum information is very sensitive to noise, which brings in errors. These errors can be avoided by introducing redundancies, but that requires making the computers larger, which brings in more problems.

Last year, IBM claimed they had done something useful with a noisy quantum computer, which was celebrated in the New York Times.

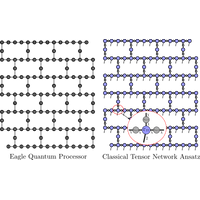
They used a noisy quantum processor called the Eagle processor with 127-qubits to perform a calculation on a 2D Trotterized Transverse-field Ising model. The Ising model is a model of coupled quantum spins which models the behaviour of tiny magnets interacting with each other, and the Trotterized transverse-filed means it is a special version. Despite the imperfections of the quantum computer, this breakthrough suggests that it could still be useful.

However, a new paper published by scientists has put IBM's achievement into doubt.

Efficient Tensor Network Simulation of IBM’s Eagle Kicked Ising Experiment

Improved classical methods for Hamiltonian simulation further shift the threshold for quantum utility.

PRX QuantumJoseph Tindall

The authors of the paper found a way to do the calculation on a conventional computer and managed to do it faster and better than IBM could on their noisy computer. They found that conventional computers outperformed the quantum computer on this task.

The study demonstrated the effectiveness of noise-mitigation techniques in quantum simulations. Using a 127-qubit system, researchers achieved more accurate results than classical tensor network methods, even with fewer resources.

This is not the first time a group has claimed to have done something spectacular on a quantum computer, only for another group to do it on a conventional computer. The most prominent example was probably Google's 2019 claim that they had proved a "quantum advantage." Back then, scientists from Google published a paper in Nature claiming that they had done a calculation on a 53-qubit quantum computer, which would have taken 10,000 years on a conventional computer. However, this claim was swiftly questioned by IBM, and it was later done by a Chinese group on a conventional computer in only five minutes.

In conclusion, while quantum computing has the potential to revolutionize various industries, there are still practical problems that need to be addressed. Although some calculations can be performed faster by quantum computers, not all calculations can be. Additionally, quantum links are incredibly fragile and susceptible to errors. While there have been claims of quantum computing achievements, there have also been instances where conventional computers outperformed quantum computers on certain tasks. Therefore, while the hype surrounding quantum computing is justified to some extent, it is important to approach the field with a critical eye and acknowledge the limitations of the technology.